Linear Algebra: Intersecting lines in the Plane - System of Equations

The purpose of these activities is to lead to the connection between
- geometric intersection of two lines in the plane and
- Gaussian algebraic method (more precisely Gauss-Jordan reduction) of finding the solution of the system of linear equations representing these lines.

So we inquire a system of two equations of type

Name:
Student Number:
Email address:

1. Intersecting lines and coordinate axis

Activity 1
This page requires a Java capable browser. Here beside you see two intersecting lines and their intersections with the coordinate axes.
You can turn the lines around by using the blue and red points and you also see the intersections with the coordinate axes.
a) Determine the coordinates of the intersection point:
b) Explain how you found the coordinates:

2. Intersecting lines and the system Activity 2
This page requires a Java capable browser. Here beside you see two intersecting lines and the corresponding system of equations.
You can turn the lines around by using the blue and red points and you also see the the corresponding equations
a) Determine the coordinates of the intersection point:
b) Explain how you found the coordinates:

3. Intersecting lines and coefficients of the system Activity 3
This page requires a Java capable browser. Here beside you see two intersecting lines and the corresponding system of equations.
Phase 1. You can control the values A1 and A2, which affect the elementary operations in the following way:
Phase 2 for reducing the first phase result in the middle of the upper part of the figure:
Use the numbers B1 ja B2 on the second real line. B1 is used now for subtracting the second equation from the first and B2 for multiplying the second.
a) Determine the coordinates of the intersection point:
b) Explain how you found the coordinates:

4. The coefficients of the system as parameters Activity 4
This page requires a Java capable browser. Here beside you see two intersecting lines and the corresponding system of equations

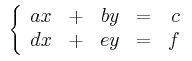
You can change the coefficients of the system (open with the Button Coefficients) and, in principle at least,
to solve systems "graphically", by using the Gauss-Jordan procedure.
a) Solve the system of equations:
The coordinates of the intersection (= the solution):
b) Explain how you found the coordinates:

Student Feedback

Here we ask you to tell what do you think about this kind of Problems and working on this kind of worksheet.



Anyone interested in this kind of worksheets may contact Martti.Pesonen(cat tail)uef.fi
Updated 10.3.2013